The Born-Mayer-Huggins problem

LAMMPS LAMMPS General Discussion
,
1

Hi Lammps users,

I’m trying to Simulate the reaction between K₂O and SiO₂ at 550°C.Compile the Born-Mayer-Huggins (BMH) potential function based on the article https://www.sciencedirect.com/science/article/pii/S0022309324000243?via%3Dihub, but encounter issues with atom loss and excessive pressure. Seeking assistance.
My data file and the input file are as follows,
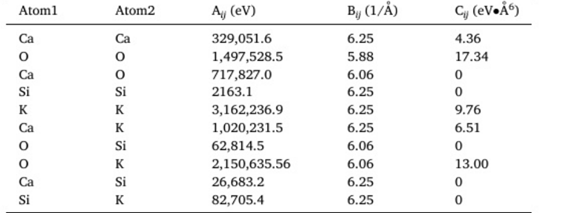
image

image
image990×265 10.8 KB

Input

units metal
boundary p p p
atom_style charge
dimension 3
timestep 0.001
neighbor 2.0 bin
neigh_modify delay 0 every 1 check yes
variable Time equal step*dt
#------------ system defination-------
read_data model.data
set type 1 charge 4.0
set type 2 charge -2.0
set type 3 charge 1.0
#----------------- setup-------
pair_style buck/coul/long 10.0
pair_coeff 1 1 2163.10000 6.25 0.0 # Si-Si
pair_coeff 2 1 62814.5000 6.06 0.0 # O-Si
pair_coeff 2 2 1497528.50 5.88 17.34 # O-O
pair_coeff 2 3 2150635.56 6.06 13.0 # O-K
pair_coeff 3 3 3162236.90 6.25 9.76 # K-K
pair_coeff 1 3 82705.4000 6.25 0.0 # Si-K
kspace_style ewald 1.0e-4

------------------------ Enegy minimization ---------------------------------------------------------------------------------------#

thermo 100
thermo_style custom step temp etotal ke pe press
dump All all custom 100 All_atom_823K.lammpstrj id type element x y z
dump_modify All element Si O K
dump_modify All sort id
min_style cg
minimize 1.0e-4 1.0e-6 100 1000
velocity all create 300 825333 mom yes rot yes dist gaussian
fix 1 all nvt temp 300 823 0.1
run 800000
Data file

HEADER

    9516  atoms
       3  atom types

  0.000000000000      56.500000000000  xlo xhi
  0.000000000000      56.560000000000  ylo yhi
  0.000000000000      65.990000000000  zlo zhi

Masses

        1   28.08500000             # Si
        2   15.99900000             # O
        3   39.09830000             # K

Atoms # charge

     1    1   4.000000        5.591000000000      52.428000000000       3.223000000000
     2    2   0.000000       54.206000000000       1.874000000000       2.132000000000
     3    2   0.000000        0.774000000000      18.322000000000      11.283000000000
     4    2   0.000000       20.249000000000      12.136000000000       4.812000000000
     5    2   0.000000       13.112000000000       6.979000000000      15.471000000000
     6    1   4.000000       47.935000000000       1.309000000000       9.889000000000
     7    2   0.000000        8.386000000000       4.478000000000      13.859000000000
     8    2   0.000000       14.469000000000      37.099000000000       5.509000000000
     9    2   0.000000       16.310000000000      18.894000000000      11.248000000000
    10    1   4.000000       41.153000000000      24.504000000000       7.955000000000
    11    2   0.000000       52.886000000000       9.634000000000       5.602000000000
    12    2   0.000000        1.640000000000      11.281000000000      11.979000000000
    13    2   0.000000       23.265000000000      12.014000000000      10.270000000000
    14    1   4.000000        9.151000000000      51.948000000000      17.128000000000
    15    2   0.000000       49.917000000000       6.020000000000      19.471000000000
    16    2   0.000000       54.204000000000       0.854000000000       5.557000000000

…………

2

As your log shows, the energy minimisation ran for 100 steps, which is also the the maximum number of steps that you set – and therefore there’s every chance that you haven’t successfully resolved atom clashes with high potential energy resulting in an immediate box explosion.

3

That kind of behavior usually indicates that your initial geometry is bad and that you have overlapping atoms. The BMH style potentials are notorious for not having a steep repulsion when atoms come too close and then lead to a “Coulomb Catastrophe” since the charges become the dominant force and anions will be pulled on top of cations and vice versa and from there on there is no salvaging the simulation. Thus you have to be extremely careful with your geometry and running a minimization cannot “fix” things like it is possible with Lennard-Jones or Morse potentials when using them in combination with Coulomb interactions.

1 Like